Facilities Sand Management: Calculations – Particle Settling Rate (B-FSM024)

Sand settles throughout oil and gas facilities. Particles settle in any low velocity zones, such as piping runs, 2 or 3 phase separators, accumulator vessels, tanks, or any other place you don’t want them!\
Calculation of settling rate is fundamentally a complex task – and the goal with this article is to provide some guidelines on basic calculations. For a full treatise of this topic see the references below. (Warning: Reference 1 by Clift, Grace, and Weber is a very detailed scientific treatise on this subject and can be difficult to interpret. Perry’s provides a good summary.)
The information below is an introduction to particle settling with the following basis;
- Free particle settling (i.e. single particle settling in still liquid). Hindered settling will be covered in a future article on desander particle transfer (SPE publication in process).
- Settling of sand particle in water. This assumes downward movement of the dense sand particle through a viscous, less-dense liquid.
- Limited discussion on drag coefficient (CD) and its derivation – only as needed to show calculation of settling rates
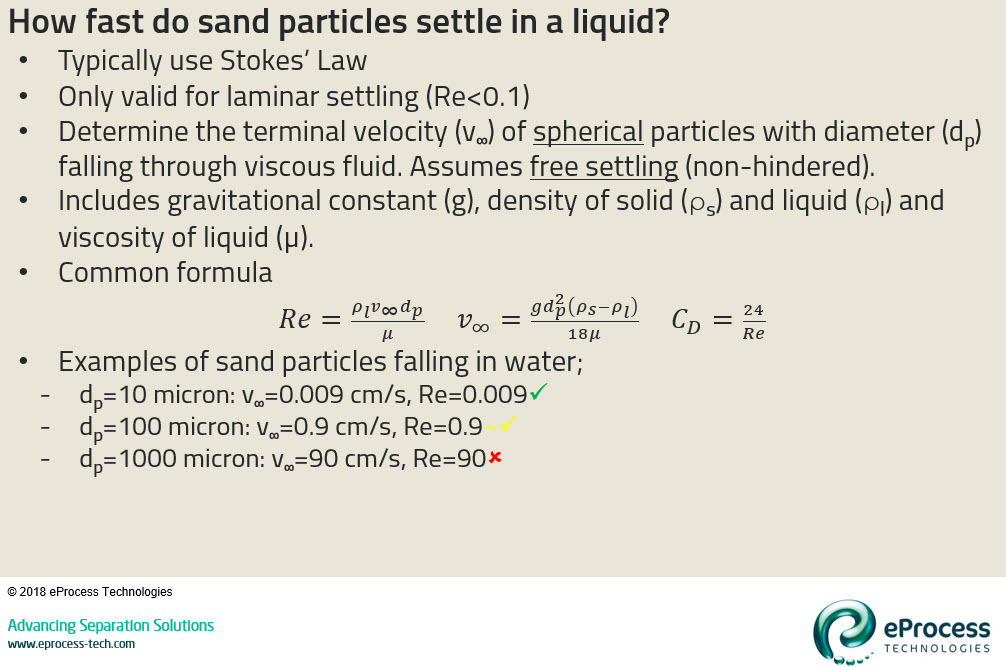
Laminar Settling: Stokes’ Law (i.e. very small round particles)
Engineers, at least in my industry, like to quote Stokes’ Law. It is a simple formula based on understandable parameters. However, it has limited use – which I see violated frequently.
- Stokes’ Law is only valid in the laminar settling region. This is the region where Reynolds Number (Re) is <0.1. With sand in water that is nominally for particles <50 microns (calculation steps below).
- This is a recursive calculation, as Re depends on settling velocity, and settling velocity validity depends on Re.
- Stokes’ Law determines the terminal settling velocity (v∞) of spherical particles with diameter (dp) falling through viscous fluid. Assumes free-settling (non-hindered).
- The calculation includes gravitational constant (g), density of solid (ρs) and liquid (ρl) and viscosity of liquid (µ).
- The hardest part of using this formula is keeping the units consistent!
- The figure below shows the formulas for Re, v∞, and CD
- Calculated values for sand of various particle sizes in water are shown in the example. Stokes’ Law is valid for 10 micron sand in water, and somewhat valid for 100 micron sand, but 1000 micron particles will deviate significantly.
Example:
Q: What is the settling rate of 50 µm sand settling in water using Stokes’ Law? Is the equation valid for this particle size? Assumptions: Sand (2650 kg/m³ density) with spherical shape settling in water (1000 kg/m³ density and 1 cP = 0.01 Pa·s viscosity).
- v∞=(9.81 m/s²)*(50×10-6m)²(2650-1000 kg/m³)/(18*0.01 Pa·s) = 2.25×10-4 m/s = 0.022 cm/s
- Re=(1000 kg/m³)(2.25×10-4 m/s)(50×10-6 m)/(0.01 Pa·s)=0.11
A: Yes, I would say that a 50 micron spherical particle is right at the limit of validity
Turbulent Settling: Large Particles
Settling rate of large spherical particles in the laminar-turbulent (0.2<Re<500) and fully turbulent (500<Re<200,000) regimes are provided in the graphic below. These relationships use most of the same terms (solid and liquid density and particle diameter), however viscosity is not present (in the turbulent formula) and the dependency on particle diameter is a square root relationship. In the turbulent regime the CD is nearly constant (i.e. independent of viscosity or particle shape).
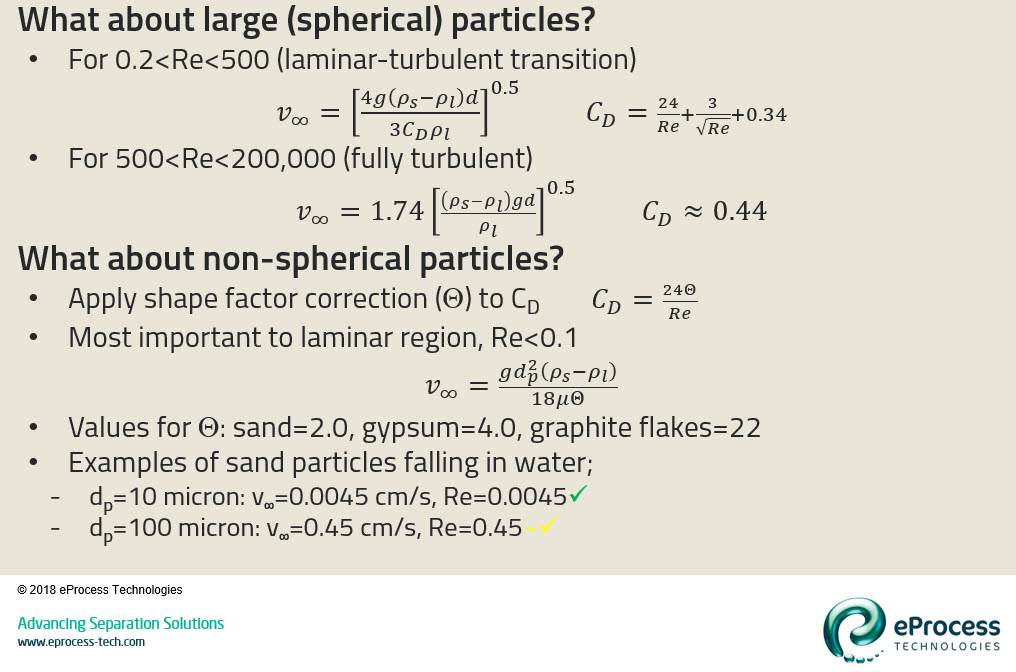
Non-Spherical Particles
This same graphic shows how to apply these relationships for non-spherical particles. All sand from a natural source has a non-spherical shape, thus this correction is important to calculate a more accurate settling velocity.
A shape factor (θ) correction is applied to the coefficient of drag. The shape factor is dependent on particle morphology (see article B-FSM-015) and some approximate values are listed. Natural sand has θ~2, while gypsum (monoclinic crystals) has θ~4. Flat flakes of graphite have a shape factor of 22, thus will settle at a rate vastly different from spherical particles.
The corrected Stokes’ Law is shown, along with comparison of settling rates for 10 and 100 micron particles. No shape factor correction is applied in the turbulent regime.
The next article will cover calculations pertaining to oil coating on sand particles.
References:
- Clift, R., Grace, J., and Weber, M.E. 2005. Bubbles, Drops, and Particles. Dover Publications, Mineola, New York.
- Green, D.W. and Perry, R.H. 2008. Perry’s Chemical Engineers’ Handbook, 8th Edition. New York: McGraw-Hill. (see pages 6-51 to 6-56 on Particle Dynamics)
